 login/create account
login/create account
Hamiltonian cycles in line graphs of infinite graphs ★★
Author(s): Georgakopoulos
Conjecture
- \item If
 is a 4-edge-connected locally finite graph, then its line graph is hamiltonian. \item If the line graph
is a 4-edge-connected locally finite graph, then its line graph is hamiltonian. \item If the line graph 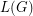 of a locally finite graph
of a locally finite graph  is 4-connected, then
is 4-connected, then 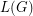 is hamiltonian.
is hamiltonian. Keywords: hamiltonian; infinite graph; line graphs
Hamiltonian cycles in line graphs ★★★
Author(s): Thomassen
Conjecture Every 4-connected line graph is hamiltonian.
Keywords: hamiltonian; line graphs
Infinite uniquely hamiltonian graphs ★★
Author(s): Mohar
Problem Are there any uniquely hamiltonian locally finite 1-ended graphs which are regular of degree 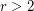 ?
?
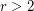 ?
? Keywords: hamiltonian; infinite graph; uniquely hamiltonian
r-regular graphs are not uniquely hamiltonian. ★★★
Author(s): Sheehan
Conjecture If  is a finite
is a finite  -regular graph, where
-regular graph, where 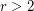 , then
, then  is not uniquely hamiltonian.
is not uniquely hamiltonian.
 is a finite
is a finite  -regular graph, where
-regular graph, where 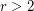 , then
, then  is not uniquely hamiltonian.
is not uniquely hamiltonian. Keywords: hamiltonian; regular; uniquely hamiltonian
Rainbow AP(4) in an almost equinumerous coloring ★★
Author(s): Conlon
Problem Do 4-colorings of 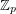 , for
, for  a large prime, always contain a rainbow
a large prime, always contain a rainbow  if each of the color classes is of size of either
if each of the color classes is of size of either 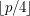 or
or  ?
?
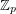 , for
, for  a large prime, always contain a rainbow
a large prime, always contain a rainbow  if each of the color classes is of size of either
if each of the color classes is of size of either 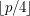 or
or  ?
? Keywords: arithmetic progression; rainbow

 Drupal
Drupal CSI of Charles University
CSI of Charles University