 login/create account
login/create account
Hamiltonian cycles in line graphs
Conjecture Every 4-connected line graph is hamiltonian.
- \item It is known that if
 is 4-edge-connected, then its line graph
is 4-edge-connected, then its line graph 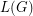 is hamiltonian. \item Thomassen's is a special case of a conjecture due to Matthews and Sumner: every 4-connected claw-free graph is hamiltonian. \item However, by a result of Ryjacek [R] conjectures of Thomassen and of Matthews and Sumner are equivalent. \item Moreover [R], one may restrict to 4-connected line graphs of triangle-free graphs.
is hamiltonian. \item Thomassen's is a special case of a conjecture due to Matthews and Sumner: every 4-connected claw-free graph is hamiltonian. \item However, by a result of Ryjacek [R] conjectures of Thomassen and of Matthews and Sumner are equivalent. \item Moreover [R], one may restrict to 4-connected line graphs of triangle-free graphs. Bibliography
[R] Zdenek Ryjacek: On a closure concept in claw-free graphs. J. Combin. Theory Ser. B 70 (1997), no. 2, 217--224, MathSciNet
*[T] Carsten Thomassen, Reflections on graph theory, J. Graph Theory 10 (1986) 309-324, MathSciNet
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University