 login/create account
login/create account
Are vertex minor closed classes chi-bounded? ★★
Author(s): Geelen
Question Is every proper vertex-minor closed class of graphs chi-bounded?
Keywords: chi-bounded; circle graph; coloring; vertex minor
Graphs with a forbidden induced tree are chi-bounded ★★★
Author(s): Gyarfas
Say that a family 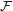 of graphs is
of graphs is 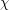 -bounded if there exists a function
-bounded if there exists a function 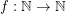 so that every
so that every  satisfies
satisfies 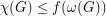 .
.
Conjecture For every fixed tree  , the family of graphs with no induced subgraph isomorphic to
, the family of graphs with no induced subgraph isomorphic to  is
is 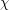 -bounded.
-bounded.
 , the family of graphs with no induced subgraph isomorphic to
, the family of graphs with no induced subgraph isomorphic to  is
is 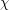 -bounded.
-bounded. Keywords: chi-bounded; coloring; excluded subgraph; tree
Asymptotic Distribution of Form of Polyhedra ★★
Author(s): Rüdinger
Problem Consider the set of all topologically inequivalent polyhedra with  edges. Define a form parameter for a polyhedron as
edges. Define a form parameter for a polyhedron as 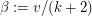 where
where  is the number of vertices. What is the distribution of
is the number of vertices. What is the distribution of 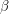 for
for 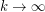 ?
?
 edges. Define a form parameter for a polyhedron as
edges. Define a form parameter for a polyhedron as 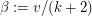 where
where  is the number of vertices. What is the distribution of
is the number of vertices. What is the distribution of 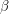 for
for 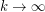 ?
? Keywords: polyhedral graphs, distribution

 Drupal
Drupal CSI of Charles University
CSI of Charles University