 login/create account
login/create account
Lucas Numbers Modulo m ★★
Author(s):
Conjecture The sequence {L(n) mod m}, where L(n) are the Lucas numbers, contains a complete residue system modulo m if and only if m is one of the following: 2, 4, 6, 7, 14, 3^k, k >=1.
Keywords: Lucas numbers
Erdős–Straus conjecture ★★
Conjecture
For all 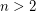 , there exist positive integers
, there exist positive integers  ,
, 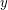 ,
,  such that
such that 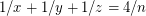 .
.
Keywords: Egyptian fraction
3 is a primitive root modulo primes of the form 16 q^4 + 1, where q>3 is prime ★★
Author(s):
Keywords:
Graph product of multifuncoids ★★
Author(s): Porton
Conjecture Let 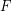 is a family of multifuncoids such that each
is a family of multifuncoids such that each 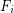 is of the form
is of the form  where
where  is an index set for every
is an index set for every 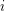 and
and 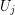 is a set for every
is a set for every 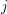 . Let every
. Let every 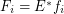 for some multifuncoid
for some multifuncoid 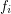 of the form
of the form 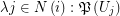 regarding the filtrator
regarding the filtrator  . Let
. Let  is a graph-composition of
is a graph-composition of 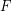 (regarding some partition
(regarding some partition  and external set
and external set 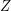 ). Then there exist a multifuncoid
). Then there exist a multifuncoid 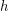 of the form
of the form 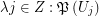 such that
such that 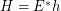 regarding the filtrator
regarding the filtrator 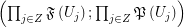 .
.
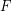 is a family of multifuncoids such that each
is a family of multifuncoids such that each 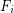 is of the form
is of the form  where
where  is an index set for every
is an index set for every 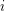 and
and 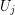 is a set for every
is a set for every 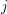 . Let every
. Let every 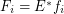 for some multifuncoid
for some multifuncoid 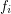 of the form
of the form 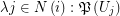 regarding the filtrator
regarding the filtrator  . Let
. Let  is a graph-composition of
is a graph-composition of 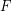 (regarding some partition
(regarding some partition  and external set
and external set 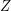 ). Then there exist a multifuncoid
). Then there exist a multifuncoid 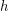 of the form
of the form 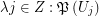 such that
such that 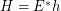 regarding the filtrator
regarding the filtrator 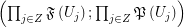 .
. Keywords: graph-product; multifuncoid
Atomicity of the poset of multifuncoids ★★
Author(s): Porton
Conjecture The poset of multifuncoids of the form 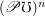 is for every sets
is for every sets  and
and  :
:
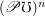 is for every sets
is for every sets  and
and  :
:- \item atomic; \item atomistic.
See below for definition of all concepts and symbols used to in this conjecture.
Refer to this Web site for the theory which I now attempt to generalize.
Keywords: multifuncoid
Atomicity of the poset of completary multifuncoids ★★
Author(s): Porton
Conjecture The poset of completary multifuncoids of the form 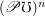 is for every sets
is for every sets  and
and  :
:
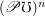 is for every sets
is for every sets  and
and  :
:- \item atomic; \item atomistic.
See below for definition of all concepts and symbols used to in this conjecture.
Refer to this Web site for the theory which I now attempt to generalize.
Keywords: multifuncoid

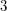 is a
is a 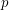 for all primes
for all primes 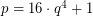 , where
, where 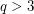 is prime.
is prime.  Drupal
Drupal CSI of Charles University
CSI of Charles University