 login/create account
login/create account
Antidirected trees in digraphs ★★
Author(s): Addario-Berry; Havet; Linhares Sales; Reed; Thomassé
An antidirected tree is an orientation of a tree in which every vertex has either indegree 0 or outdergree 0.
Conjecture Let  be a digraph. If
be a digraph. If 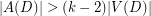 , then
, then  contains every antidirected tree of order
contains every antidirected tree of order  .
.
 be a digraph. If
be a digraph. If 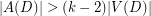 , then
, then  contains every antidirected tree of order
contains every antidirected tree of order  .
. Keywords:
Decomposing an even tournament in directed paths. ★★★
Author(s): Alspach; Mason; Pullman
Conjecture Every tournament  on an even number of vertices can be decomposed into
on an even number of vertices can be decomposed into  directed paths.
directed paths.
 on an even number of vertices can be decomposed into
on an even number of vertices can be decomposed into  directed paths.
directed paths. Keywords:
Oriented trees in n-chromatic digraphs ★★★
Author(s): Burr
Conjecture Every digraph with chromatic number at least 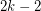 contains every oriented tree of order
contains every oriented tree of order  as a subdigraph.
as a subdigraph.
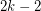 contains every oriented tree of order
contains every oriented tree of order  as a subdigraph.
as a subdigraph. Keywords:

 Drupal
Drupal CSI of Charles University
CSI of Charles University