 login/create account
login/create account
 -degenerate graph with chromatic number
-degenerate graph with chromatic number 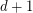 and girth
and girth  , for all
, for all 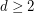 and
and 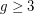 ?
? A graph is  -degenerate if every subgraph has a vertex of degree at most
-degenerate if every subgraph has a vertex of degree at most  . Such graphs are
. Such graphs are 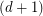 -colourable by a minimum-degree-greedy algorithm. This bound is tight for complete graphs. More interestingly, Alon-Krivelevich-Sudakov [AKS] proved that it is tight for triangle-free graphs. That is, there are
-colourable by a minimum-degree-greedy algorithm. This bound is tight for complete graphs. More interestingly, Alon-Krivelevich-Sudakov [AKS] proved that it is tight for triangle-free graphs. That is, there are  -degenerate triangle-free graphs with chromatic number
-degenerate triangle-free graphs with chromatic number 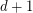 . Thus the answer to the above question is `yes' for
. Thus the answer to the above question is `yes' for 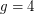 . Odd cycles show that the answer is `yes' for
. Odd cycles show that the answer is `yes' for 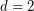 and
and 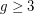 . A `yes' answer for
. A `yes' answer for 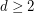 and
and  would generalise the famous result of Erdős [E], who proved that there are
would generalise the famous result of Erdős [E], who proved that there are  -chromatic graphs with girth
-chromatic graphs with girth  for all
for all  and
and  . A `no' answer would also be interesting---this would provide a non-trivial upper bound on the chromatic number of
. A `no' answer would also be interesting---this would provide a non-trivial upper bound on the chromatic number of  -degenerate graphs with girth
-degenerate graphs with girth  .
.
Bibliography
[AKS] Noga Alon, Michael Krivelevich, and Benny Sudakov. Coloring graphs with sparse neighborhoods. J. Combin. Theory Ser. B 77.1:73--82, 1999.
[E] Paul Erdős, Graph theory and probability, Canad. J. Math. 11:34--38, 1959.
*[W] David R. Wood, Hypergraph Colouring and Degeneracy, arXiv:1310.2972, 2013.
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University