 login/create account
login/create account
Choice number of complete multipartite graphs with parts of size 4 (Solved)
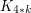 for general
for general  ?
?
For positive integers  and
and  , let
, let  be the complete
be the complete  -partite graph in which every part has size
-partite graph in which every part has size  . For the definition of the choice number (list chromatic number) see the Wikipedia page.
. For the definition of the choice number (list chromatic number) see the Wikipedia page.
Determining the choice number of  seems to be a natural first step to obtaining a general bound on the choice number of graphs on a bounded number of vertices.
seems to be a natural first step to obtaining a general bound on the choice number of graphs on a bounded number of vertices.
An old result of Erdos, Rubin and Taylor [ERT80] is that the choice number of 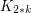 is
is  . Ohba [Ohb02] conjectured a generalization of this result which was proved by Noel, Reed and Wu [NRW12] (also see [Noe13]): if
. Ohba [Ohb02] conjectured a generalization of this result which was proved by Noel, Reed and Wu [NRW12] (also see [Noe13]): if 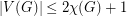 , then the choice number of
, then the choice number of  is
is 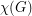 .
.
Kierstead [Kier00] proved that the choice number of 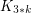 is exactly
is exactly 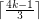 . The upper bound was generalized by Noel, West, Wu and Zhu [NWWZ13] to the following: if
. The upper bound was generalized by Noel, West, Wu and Zhu [NWWZ13] to the following: if 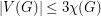 , then the choice number of
, then the choice number of  is at most TeX Embedding failed!.}
is at most TeX Embedding failed!.}
More generally, Noel et al. [NWWZ13] proved that, for any graph  , the choice number of
, the choice number of  is at most
is at most 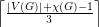 (also see [Noe13]). This implies the following:
(also see [Noe13]). This implies the following:
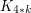 is at most
is at most 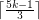 .
. A lower bound on the choice number of 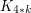 is given by Yang [Yan03]:
is given by Yang [Yan03]:
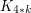 is at least
is at least 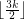 .
. The problem was recently solved by Kierstead, Salmon and Wang [KSW14]. They proved that, surprisingly, the lower bound 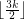 is tight for all
is tight for all  .
.
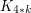 is equal to
is equal to 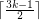 .
. Naturally, we wonder whether this result can be generalised to all graphs on at most 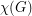 vertices. See this posting as well.
vertices. See this posting as well.
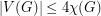 , then
, then 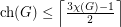 ?
? Bibliography
[ERT80] P. Erdos, A. L. Rubin, and H. Taylor. Choosability in graphs. Congress. Numer., XXVI, pages 125–157, 1980.
[Kie00] H. A. Kierstead. On the choosability of complete multipartite graphs with part size three. Discrete Math., 211(1-3):255–259, 2000.
[KSW14] H. A. Kierstead, A. Salmon and R. Wang. On the Choice Number of Complete Multipartite Graphs With Part Size Four.
[Noe13] J. A. Noel. Choosability of Graphs With Bounded Order: Ohba's Conjecture and Beyond. Master's thesis, McGill University, Montreal. pdf
[NRW12] J. A. Noel, B. A. Reed, and H. Wu. A Proof of a Conjecture of Ohba. Preprint, arXiv:1211.1999v1, November 2012. Webpage
[NWWZ13] J. A. Noel, D. B. West, H. Wu, and X. Zhu. Beyond Ohba's Conjecture: A bound on the choice number of  -chromatic graphs with
-chromatic graphs with  vertices. Preprint, arXiv:1308.6739v1, August 2013. pdf
vertices. Preprint, arXiv:1308.6739v1, August 2013. pdf
[Ohb02] K. Ohba. On chromatic-choosable graphs. J. Graph Theory, 40(2):130–135, 2002.
[Yan03] D. Yang. Extension of the game coloring number and some results on the choosability of complete multipartite graphs. PhD thesis, Arizona State University, Tempe, Arizona, 2003.
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University