 login/create account
login/create account
 has a proper
has a proper 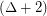 -edge-colouring so that every cycle contains edges of at least three distinct colours.
-edge-colouring so that every cycle contains edges of at least three distinct colours. An edge-colouring with the property that every cycle contains edges of at least three distinct colours is called an acyclic edge-colouring. It is known (see [AMR]) that every graph of maximum degree  has an acyclic edge-colouring of size
has an acyclic edge-colouring of size 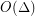 . The best upper bound so far is
. The best upper bound so far is  and is due to Esperet and Parreau [EP]. It is also known (see [ASZ]) that this conjecture is true for graphs with girth at least
and is due to Esperet and Parreau [EP]. It is also known (see [ASZ]) that this conjecture is true for graphs with girth at least 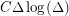 (for some fixed constant
(for some fixed constant  ).
).
Bibliography
[AMR] N. Alon, C. McDiarmid and B. Reed, Acyclic colouring of graphs, Random Structures and Algorithms 2 (1991), 277-288. MathSciNet
[ASZ] N. Alon, B. Sudakov and A. Zaks, Acyclic edge-colorings of graphs, J. Graph Theory 37 (2001), 157-167. MathSciNet
[EP] L. Esperet and A. Parreau, Acyclic edge-coloring using entropy compression, arXiv:1206.1535 [math.CO].
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University