 login/create account
login/create account
Chudnovsky, Maria
Non-edges vs. feedback edge sets in digraphs ★★★
Author(s): Chudnovsky; Seymour; Sullivan
For any simple digraph  , we let
, we let 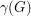 be the number of unordered pairs of nonadjacent vertices (i.e. the number of non-edges), and
be the number of unordered pairs of nonadjacent vertices (i.e. the number of non-edges), and 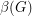 be the size of the smallest feedback edge set.
be the size of the smallest feedback edge set.
Conjecture If  is a simple digraph without directed cycles of length
is a simple digraph without directed cycles of length  , then
, then  .
.
 is a simple digraph without directed cycles of length
is a simple digraph without directed cycles of length  , then
, then  .
. Keywords: acyclic; digraph; feedback edge set; triangle free

 Drupal
Drupal CSI of Charles University
CSI of Charles University