Home » Subject » Topology
Importance: Medium ✭✭
Author(s): Porton, Victor
Subject: Topology
Keywords: funcoid
inward reloid
outward reloid
reloid
Recomm. for undergrads: no
Posted by: porton
on: August 9th, 2007
Solved by: Porton, Victor
Conjecture   For any funcoid $ f $ and reloid $ g $ having the same source and destination \[ ( \mathsf{\tmop{RLD}})_{\tmop{out}} f \subseteq g \subseteq ( \mathsf{\tmop{RLD}})_{\tmop{in}} f \Leftrightarrow ( \mathsf{\tmop{FCD}}) g = f. \]

See Algebraic General Topology for definitions of used concepts.

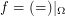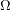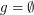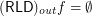Counter-example: $ f=(=)|_{\Omega} $ where $ \Omega $ is the Frechet filter, $ g=\emptyset $. Then $ (\mathsf{\tmop{RLD}})_{\tmop{out}} f=\emptyset $.

Related problems

Funcoid corresponding to inward reloid

Bibliography

*Victor Porton. Algebraic General Topology


* indicates original appearance(s) of problem.
1 comment

Reply

Comments are limited to a maximum of 1000 characters.
More information about formatting options