Home » Subject » Combinatorics
Importance: Low ✭
Author(s): Gonshor, Harry
Subject: Combinatorics
Keywords: surreal numbers
Recomm. for undergrads: yes
Posted by: Lukáš Lánský
on: April 7th, 2012
Conjecture   Every surreal number has a unique sign expansion, i.e. function $ f: o\rightarrow \{-, +\} $, where $ o $ is some ordinal. This $ o $ is the length of given sign expansion and also the birthday of the corresponding surreal number. Let us denote this length of $ s $ as $ \ell(s) $.

It is easy to prove that

$$ \ell(s+t) \leq \ell(s)+\ell(t) $$

What about

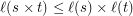
$$ \ell(s\times t) \leq \ell(s)\times\ell(t) $$

?

This is strongly conjectured to be true by Gonshor in [Gon86]. There is an easy way to prove that

$$ \ell(s\times t) \leq 3^{\ell(s)+\ell(t)} $$

Bibliography

*[Gon86] Harry Gonshor, An Introduction to the Theory of Surreal Numbers, Cambridge University Press, Cambridge, 1986.


* indicates original appearance(s) of problem.
2 comments

Reply

Comments are limited to a maximum of 1000 characters.
More information about formatting options