Home » Keyword index

order, dividing


Group Theory

Subgroup formed by elements of order dividing n ★★

Author(s): Frobenius

Conjecture  

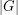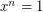Suppose $ G $ is a finite group, and $ n $ is a positive integer dividing $ |G| $. Suppose that $ G $ has exactly $ n $ solutions to $ x^{n} = 1 $. Does it follow that these solutions form a subgroup of $ G $?

Keywords: order, dividing

Posted by dlh12
updated March 30th, 2012
4 comments
Syndicate content