 login/create account
login/create account
Gernert, Dieter
The sum of the two largest eigenvalues ★★
Author(s): Gernert
Problem Let  be a graph on
be a graph on  vertices and let
vertices and let 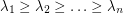 be the eigenvalues of
be the eigenvalues of  . Is
. Is 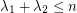 ?
?
 be a graph on
be a graph on  vertices and let
vertices and let 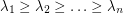 be the eigenvalues of
be the eigenvalues of  . Is
. Is 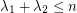 ?
? Keywords: eigenvalues; spectrum

 Drupal
Drupal CSI of Charles University
CSI of Charles University