Grotzsch proved that every triangle free (and loopless) planar graph is 3-colorable. By flow/coloring duality, this is equivalent to the statement that every 4-edge-connected planar graph has a nowhere-zero 3-flow. The 3-flow conjecture asserts that this is still true without the assumption of planarity.

Jaeger proved that 4-edge-connected graphs have nowhere-zero 4-flows, but very little is known about nowhere-zero 3-flows. In particular, the following weak version of the 3-flow conjecture is still wide open.

Conjecture  (The weak 3-flow conjecture (Jaeger))   There exists a fixed integer so that every -edge-connected graph has a nowhere-zero 3-flow.

Lai and Zhang [LZ] have proved that if has vertices and edge-connectivity at least then has a nowhere-zero 3-flow. A similar result (edge connectivity at least 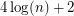) also follows from a theorem of Alon, Linial, and Meshulam [ALM] on additive bases of vector spaces.