 login/create account
login/create account
Closing Lemma for Diffeomorphism (Dynamical Systems)
Conjecture Let 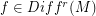 and
and 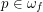 . Then for any neighborhood
. Then for any neighborhood 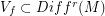 there is
there is 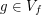 such that
such that  is periodic point of
is periodic point of 
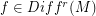 and
and 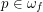 . Then for any neighborhood
. Then for any neighborhood 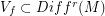 there is
there is 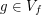 such that
such that  is periodic point of
is periodic point of 
There is an analogous conjecture for flows (  vector fields . In the case of diffeos this was proved by Charles Pugh for
vector fields . In the case of diffeos this was proved by Charles Pugh for 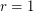 . In the case of Flows this has been solved by Sushei Hayahshy for
. In the case of Flows this has been solved by Sushei Hayahshy for 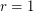 . But in the two cases the problem is wide open for
. But in the two cases the problem is wide open for 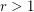
Bibliography
Dynamics beyond uniform hyperbolicity :\Springer [Encyclopaedia of Mathematical Sciences Volume 102, Mathematical Phisics,2005]
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University