 login/create account
login/create account
Algebraic independence of pi and e
Bibliography
* indicates original appearance(s) of problem.
in which subfield K of which field L?
On February 16th, 2011 Comet says:
After all, e to the pi i = -1, so this shows that pi and e are not always algebraically independent.
By definition?
On July 16th, 2011 Anonymous says:
I think any two distinct transcendental numbers must be algebraically independent, almost by definition. Since e and pi are transcendental, they must be a. i. No? - David Spector
not all transcedentials are algebraically independant
On August 5th, 2011 cubola zaruka says:
pi and 4-pi are both transcedential and sum to 4, so are not algebraically independant.
two transcendentals are not necessarily algebraically independen
On July 21st, 2011 Anonymous says:
e and e^2 are both transcendental but (e,e^2) makes the two-variable polynomial f(x,y)=x^2-y equal to zero

 and
and  are
are  Drupal
Drupal CSI of Charles University
CSI of Charles University
Schanuel's conjecture
Assuming Schanuel's conjecture, one can show that and
and  are algebraically independent over
are algebraically independent over 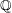 .
.