Let be the function we are integrating. Let denote the region for which and that contains the value of where . Then we note that and the sines of the integral alternate, so we can just calculate the first one and everything else will be bounded (in particular by ). With a bit of Taylor approximation, we can bound the size of each by , and noting that is always positive for , we can replace the with and then bound by . This gives us the bound we claimed above and we are done.
Solution (final part)
Let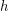 be the function we are integrating. Let
be the function we are integrating. Let 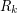 denote the region for which
denote the region for which 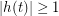 and that contains the value of
and that contains the value of  where
where 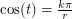 . Then we note that
. Then we note that 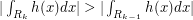 and the sines of the integral alternate, so we can just calculate the first one and everything else will be bounded (in particular by
and the sines of the integral alternate, so we can just calculate the first one and everything else will be bounded (in particular by 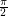 ). With a bit of Taylor approximation, we can bound the size of each
). With a bit of Taylor approximation, we can bound the size of each 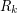 by
by ![$ \frac{4\sqrt[4]{a-1}}{\sqrt{r}} $](/files/tex/97f0b3c5d92e4c02cba0a14ade83ce9080e7d0bf.png) , and noting that
, and noting that 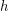 is always positive for
is always positive for 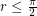 , we can replace the
, we can replace the 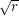 with
with  and then bound
and then bound 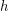 by
by 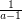 . This gives us the bound we claimed above and we are done.
. This gives us the bound we claimed above and we are done.
Jacob Steinhardt