Home » Subject » Topology
Importance: Medium ✭✭
Author(s): Porton, Victor
Subject: Topology
Keywords: hyperfuncoids
multidimensional
Recomm. for undergrads: no
Posted by: porton
on: December 9th, 2014

Let $ \mathfrak{A} $ be an indexed family of sets.

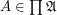Products are $ \prod A $ for $ A \in \prod \mathfrak{A} $.

Hyperfuncoids are filters 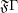$ \mathfrak{F} \Gamma $ on the lattice 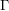$ \Gamma $ of all finite unions of products.

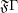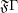Problem   Is $ \bigcap^{\mathsf{\tmop{FCD}}} $ a bijection from hyperfuncoids $ \mathfrak{F} \Gamma $ to:
    \item prestaroids on $ \mathfrak{A} $; \item staroids on $ \mathfrak{A} $; \item completary staroids on $ \mathfrak{A} $?

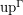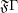If yes, is $ \operatorname{up}^{\Gamma} $ defining the inverse bijection? If not, characterize the image of the function $ \bigcap^{\mathsf{\tmop{FCD}}} $ defined on $ \mathfrak{F} \Gamma $.

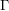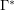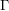Consider also the variant of this problem with the set $ \Gamma $ replaced with the set $ \Gamma^{\ast} $ of complements of elements of the set $ \Gamma $.

It's used notation from Algebraic General Topology draft book

Bibliography



* indicates original appearance(s) of problem.
add new comment

Reply

Comments are limited to a maximum of 1000 characters.
More information about formatting options