Open Problem Garden

Importance: Medium ✭✭

Author(s):

Subject:

Keywords:

Recomm. for undergrads: no

Posted	by:	porton
	on:	September 17th, 2013

Solved by:

Porton, Victor

Conjecture Every monovalued reloid is metamonovalued.

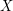Let $f$ is a monovalued reloid. Then there is a principal filter $X$ and principal monovalued reloid 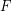$F$ such that $f = F|_X$ .

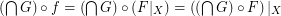
$\left( \bigcap G \right) \circ f = \left( \bigcap G \right) \circ ( F|_X) = \left( \left( \bigcap G \right) \circ F \right) |_X$

It follows that it's enough to prove it for monovalued principal reloids.

Bibliography

Solved in the new version of this book preprint: *Algebraic General Toplogy. Volume 1

* indicates original appearance(s) of problem.