 login/create account
login/create account
Conjecture Let  be a Cantor set embedded in
be a Cantor set embedded in  . Is there a self-homeomorphism
. Is there a self-homeomorphism  of
of  for every
for every  greater than
greater than  so that
so that  moves every point by less than
moves every point by less than  and
and 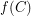 does not intersect
does not intersect  ? Such an embedded Cantor set for which no such
? Such an embedded Cantor set for which no such  exists (for some
exists (for some  ) is called "sticky". For what dimensions
) is called "sticky". For what dimensions  do sticky Cantor sets exist?
do sticky Cantor sets exist?
 be a Cantor set embedded in
be a Cantor set embedded in  . Is there a self-homeomorphism
. Is there a self-homeomorphism  of
of  for every
for every  greater than
greater than  so that
so that  moves every point by less than
moves every point by less than  and
and 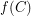 does not intersect
does not intersect  ? Such an embedded Cantor set for which no such
? Such an embedded Cantor set for which no such  exists (for some
exists (for some  ) is called "sticky". For what dimensions
) is called "sticky". For what dimensions  do sticky Cantor sets exist?
do sticky Cantor sets exist? I borrowed this conjecture from this forum thread.
Certainly I understand this conjecture wrongly:  is a subset of a line segment. Consider a homeomorphism which moves all points of
is a subset of a line segment. Consider a homeomorphism which moves all points of  orthogonally to this line segment by
orthogonally to this line segment by 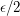 . This would be a solution of this problem. Obviously it is not what is meant.
. This would be a solution of this problem. Obviously it is not what is meant.
Indeed I submit the problem to OPG as is in the hope that somebody will correct my wrong understanding and adjust the formulation to not be misunderstood as by me.
Bibliography
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University