 login/create account
login/create account
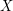 be a complex projective variety. Then every Hodge class is a rational linear combination of the cohomology classes of complex subvarieties of
be a complex projective variety. Then every Hodge class is a rational linear combination of the cohomology classes of complex subvarieties of 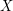 .
.
A complex projective variety is the set of zeros of a finite collection of homogeneous polynomials on projective space, and we are concerned with the singular cohomology ring. There is a well known Hodge Decomposition of the cohomology into groups 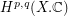 which hare holomorphic in
which hare holomorphic in  variables and antiholomorphic in
variables and antiholomorphic in 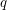 variables with the property that
variables with the property that  .
.
So we define the Hodge classes to be those in the intersection 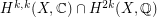 . It is fairly easy to show that the cohomology class of a subvariety is Hodge. We say that a cycle is algebraic if it is a rational linear combination of the classes of subvarieties. So every algebraic cycle is Hodge. In dimension one, we have the following result:
. It is fairly easy to show that the cohomology class of a subvariety is Hodge. We say that a cycle is algebraic if it is a rational linear combination of the classes of subvarieties. So every algebraic cycle is Hodge. In dimension one, we have the following result:
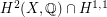 is the cohomology class of a divisor, and so is algebraic.
is the cohomology class of a divisor, and so is algebraic. It's also true that if the Hodge Conjecture holds for cycles of degree 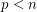 , then it holds for cycles of degree
, then it holds for cycles of degree 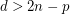 . So this and the (1,1) Theorem show that the Hodge Conjecture is true for complex curves, surfaces and threefolds.
. So this and the (1,1) Theorem show that the Hodge Conjecture is true for complex curves, surfaces and threefolds.
Bibliography
*[Hod] Hodge, W. V. D. "The topological invariants of algebraic varieties". Proceedings of the International Congress of Mathematicians, Cambridge, MA, 1950, vol. 1, pp. 181–192.
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University