 login/create account
login/create account
Singmaster, David
Singmaster's conjecture ★★
Author(s): Singmaster
Conjecture There is a finite upper bound on the multiplicities of entries in Pascal's triangle, other than the number  .
.
 .
. The number  appears once in Pascal's triangle,
appears once in Pascal's triangle,  appears twice,
appears twice,  appears three times, and
appears three times, and 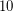 appears
appears  times. There are infinite families of numbers known to appear
times. There are infinite families of numbers known to appear  times. The only number known to appear
times. The only number known to appear  times is
times is  . It is not known whether any number appears more than
. It is not known whether any number appears more than  times. The conjectured upper bound could be
times. The conjectured upper bound could be  ; Singmaster thought it might be
; Singmaster thought it might be 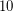 or
or 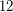 . See Singmaster's conjecture.
. See Singmaster's conjecture.
Keywords: Pascal's triangle

 Drupal
Drupal CSI of Charles University
CSI of Charles University