 login/create account
login/create account
Odd-cycle transversal in triangle-free graphs
Conjecture If  is a simple triangle-free graph, then there is a set of at most
is a simple triangle-free graph, then there is a set of at most 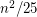 edges whose deletion destroys every odd cycle.
edges whose deletion destroys every odd cycle.
 is a simple triangle-free graph, then there is a set of at most
is a simple triangle-free graph, then there is a set of at most 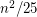 edges whose deletion destroys every odd cycle.
edges whose deletion destroys every odd cycle. Bibliography
*[EFPS] P. Erdös, R. Faudree, J. Pach and J. Spencer, How to make a graph bipartite. J. Combin. Theory Ser. B 45 (1988), 86--98.
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University